2018
Paris, France
General relativity project
The following gifs were created as part of a project realized in 2018 during my second year of the master
Dynamiques des systèmes
gravitationnels at the observatory of Paris. The project consists in computing the motion of particules around a black hole and I
numerically integrate the equations of geodesics in the Schwarzschild's metric.
The central black hole has the mass of the Sun
and the axes are in kilometers. The coordinate time was used as the affine parameter in the integrations, meaning that the motion
of the massless particules is the one seen by an asymptotic observer, that is someone at rest, located infinitely far away from the black hole,
were spacetime is flat. A Runge Kutta method of fourth order was especially coded.
The inner black circle is the event horizon of the black hole, located one Schwarzschild radius away from the singularity, or roughly 3 kilometers.
The outer black circle is the radius of the innermost stable circular orbit (ISCO), located 3 Schwarzschild radii
away from the singularity.
2018
Paris, France
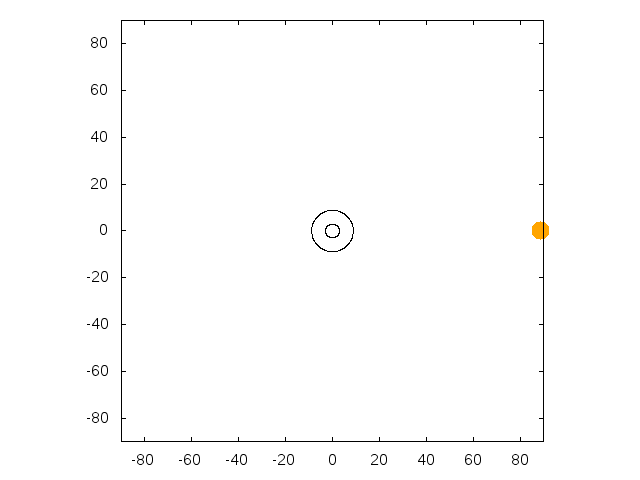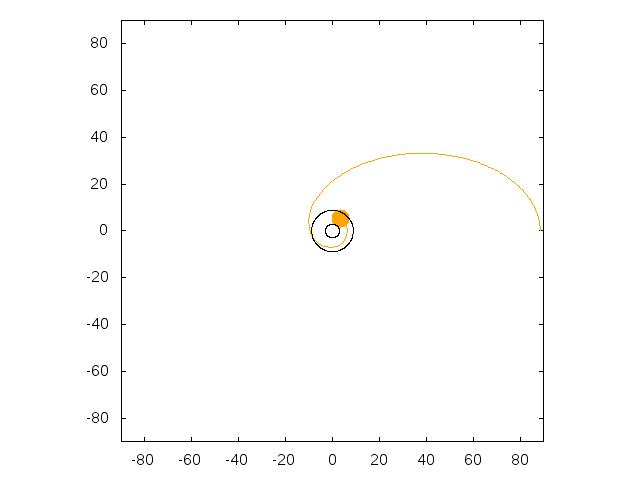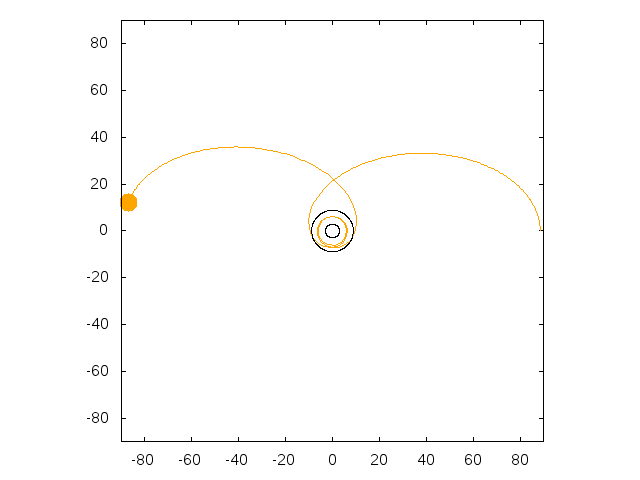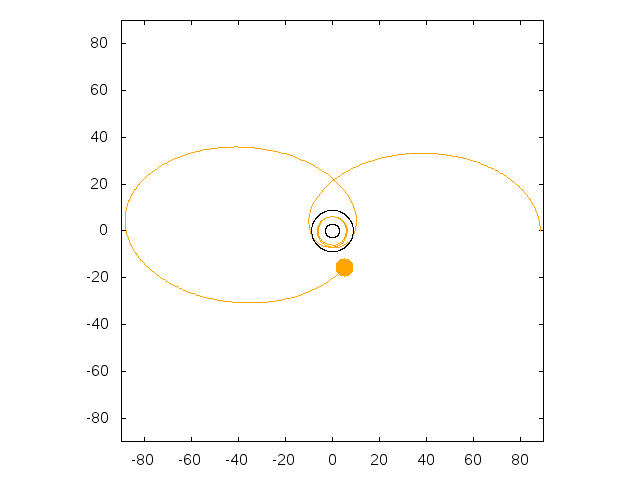
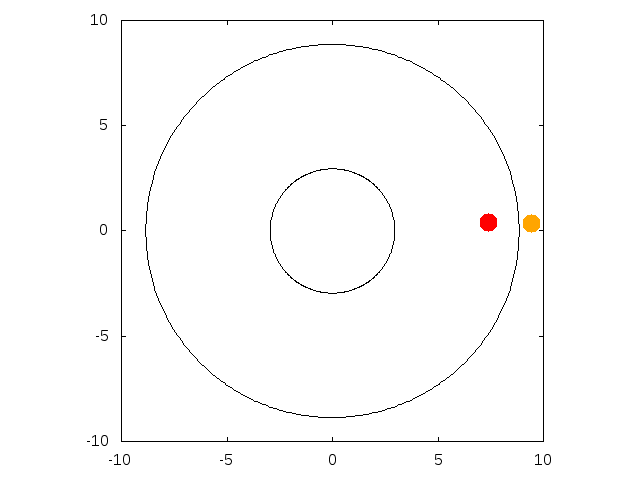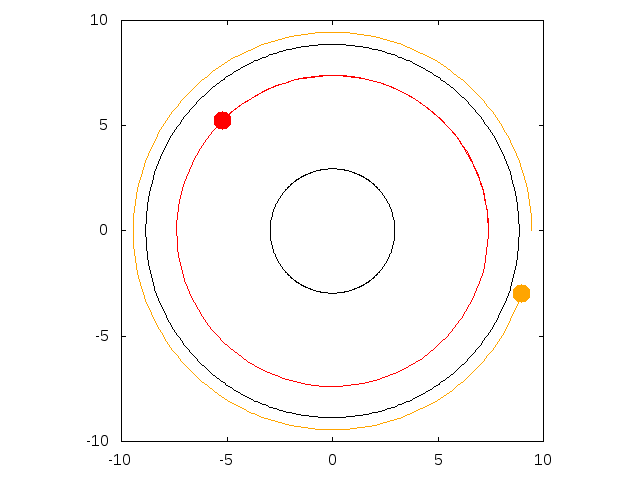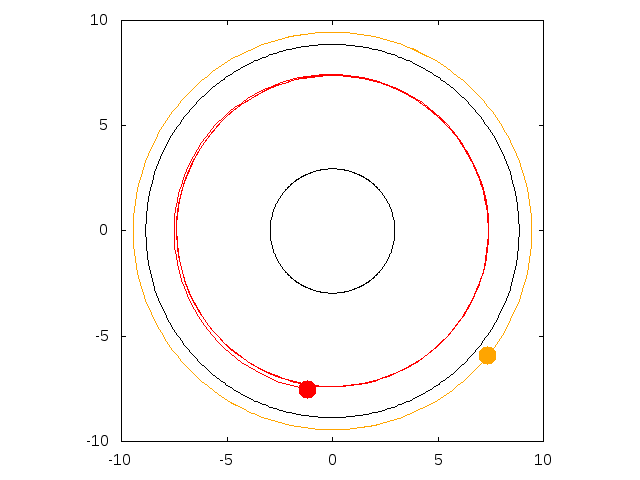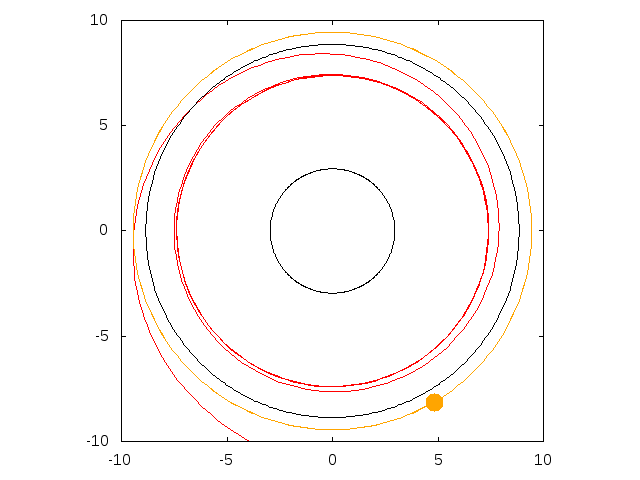
Isco
This simulation shows the existence of an Innermost Stable Circular Orbit (ISCO). While the orange particule is stable, the red particule is below the ISCO and gets ejected from its orbit. When it gets ejected, it can either fall onto the singularity, or get away from the black hole.
2018
Paris, France
Ejection from the ISCO
For orbits below 2 Schwarzschild radii, an ejected particule reaches infinity, while for orbits above, it stays bounded to the black hole. The orange particule has a 2.1 Schwarzschild radius and stays bounded while the red particule, with a 1.95 Schwarzschild radius initial orbit goes to infinity. When the apoapsis is not infinite, its value depends only on the initial radius.
2018
Paris, France
Periapsis precession
One of the first effect of general relativity described by Einstein is the precession of the orbit. Here, the particule is dropped from 30 Schwarzschild radii with an horizontal speed such that its periapsis is close to the black hole, leading to a huge precession of the periapsis.
2018
Paris, France
Capture on a circular orbit
Here, the initial speed is chosen so that the particule settles on an (unstable) circular orbit. The radius of the circular orbit is then determined only by the height of the apoapsis (here 30 Schwarzschild radii).